Pythagorean Theorem Drawing
Pythagorean Theorem Drawing - In a right triangle with sides a, b, and hypotenuse c, the theorem states that a² + b² = c². It can be shown that the results from these equations do, in fact, satisfy the pythatorean theorem: A 2 + b 2 = c 2. You can learn more about the pythagorean theorem and review its algebraic proof. A + b = c. Web pythagorean theorem in 3d. Label the point of intersection d. The pythagorean theorem formula is a² + b² = c². This fun math lesson explores right pyramids and rectangular prisms, guiding you through solving for unknown lengths by applying the theorem multiple times. Square root of both sides: Web draw a new triangle, \(\triangle def\), so that \(\angle f = 90^{\circ}\), \(d = a\), and \(e = b\) (figure \(\pageindex{4}\)). Draw a right triangle, and label the vertices a, b, and c, where c is the vertex of the right angle. Put in what we know: Designate the legs of length a and b and hypotenuse of length. To be specific, relating the two legs and the hypotenuse, the longest side. It can be shown that the results from these equations do, in fact, satisfy the pythatorean theorem: Side c c is known as the hypotenuse. Draw a line through vertex c that is perpendicular to the segment ab. A 2 + b 2 = c 2. It can be shown that the results from these equations do, in fact, satisfy the pythatorean theorem: Example \ (\pageindex {5}\) example \ (\pageindex {6}\) example \ (\pageindex {8}\) the distance formula. Example \ (\pageindex {10}\) exercise \ (\pageindex {1}\) exercise \ (\pageindex {2}\) exercise \ (\pageindex {3}\) exercise \ (\pageindex {4}\) exercise \ (\pageindex {5}\) exercise \ (\pageindex {6}\). You can use the pythagorean theorem is to find the distance between two points. Let a denote the length of ac, b denote the length of bc, and c denote the length of ab. We have \(f^2 = d^2 + e^2 = a^2 + b^2 = c^2\) and therefore \(f = c\). It can be shown that the results from. To solve the pythagorean theorem, we need to know the lengths of at least two sides of a right triangle. It works the other way around, too: A^2+b^2=c^2 a2 + b2 = c2. The pythagorean theorem describes a special relationship between the sides of a right triangle. Side c c is known as the hypotenuse. Let a denote the length of ac, b denote the length of bc, and c denote the length of ab. It states that in any right triangle, the sum of the squares of the lengths of the two legs equals the square of the length of the hypotenuse. Web the pythagorean theorem tells how the lengths of the three sides. Web the pythagorean theorem is a cornerstone of math that helps us find the missing side length of a right triangle. It works the other way around, too: Draw four congruent right triangles. This fun math lesson explores right pyramids and rectangular prisms, guiding you through solving for unknown lengths by applying the theorem multiple times. A^2+b^2=c^2 a2 + b2. Web however, one of the most famous theorems in all of mathematics does bear his name, the pythagorean theorem. Discover how to find the length of an edge in a 3d shape using the pythagorean theorem! It only works for right triangles. Finding the length of the hypotenuse. To solve the pythagorean theorem, we need to know the lengths of. Web in mathematics, the pythagorean theorem or pythagoras' theorem is a fundamental relation in euclidean geometry between the three sides of a right triangle. Web the pythagorean theorem tells how the lengths of the three sides of a right triangle relate to each other. Side c c is known as the hypotenuse. Designate the legs of length a and b. Web when m and n are both positive integers and m < n, pythagorean triples can be generated using the following equations where a and b will be the legs of the right triangle and c will be the hypotenuse. 1 + 1 = c2. \(\triangle def\) is a right triangle, so by theorem \(\pageindex{1}\), \(f^2 = d^2 + e^2\).. Example \ (\pageindex {10}\) exercise \ (\pageindex {1}\) exercise \ (\pageindex {2}\) exercise \ (\pageindex {3}\) exercise \ (\pageindex {4}\) exercise \ (\pageindex {5}\) exercise \ (\pageindex {6}\) 1 + 1 = c2. Designate the legs of length a and b and hypotenuse of length c. 12 + 12 = c2. Web the pythagorean theorem states that in a right triangle, the sum of the squares of the two shorter sides equals the square of the longest side (the hypotenuse). Draw a line through vertex c that is perpendicular to the segment ab. Web the pythagorean theorem tells how the lengths of the three sides of a right triangle relate to each other. Finding the length of a leg. Web however, one of the most famous theorems in all of mathematics does bear his name, the pythagorean theorem. According to the pythagorean theorem, the square of the hypotenuse is equal to the sum of the squares of the other two sides of a triangle. A2 + b2 = c2. This fun math lesson explores right pyramids and rectangular prisms, guiding you through solving for unknown lengths by applying the theorem multiple times. And this will be true: Web the pythagorean theorem is a cornerstone of math that helps us find the missing side length of a right triangle. Web draw a new triangle, \(\triangle def\), so that \(\angle f = 90^{\circ}\), \(d = a\), and \(e = b\) (figure \(\pageindex{4}\)). The pythagorean theorem formula is a² + b² = c².
Pythagorean Theorem Spiral Project A Fun And Educational Activity For
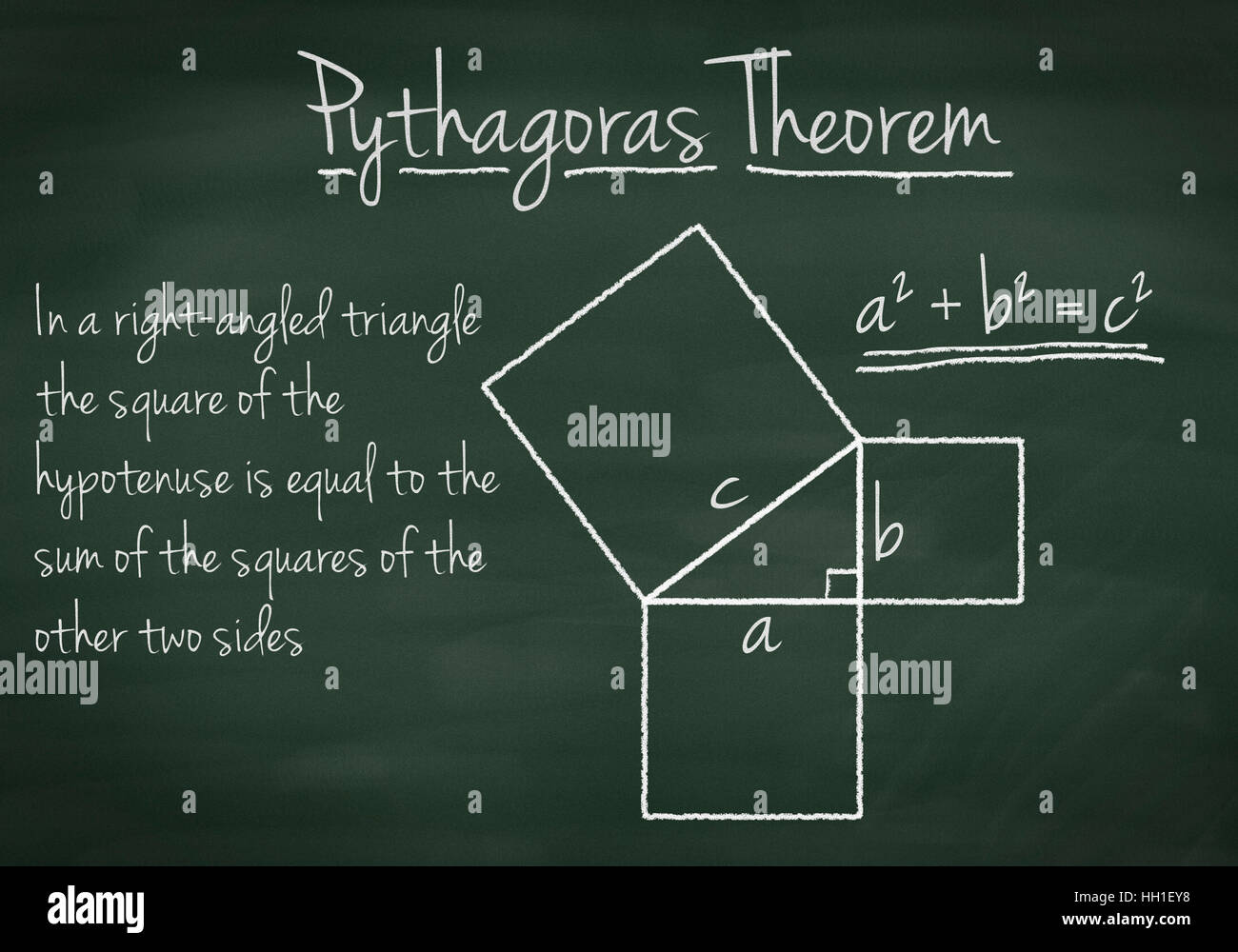
Pythagoras Theorem explained on a chalkboard Stock Photo Alamy
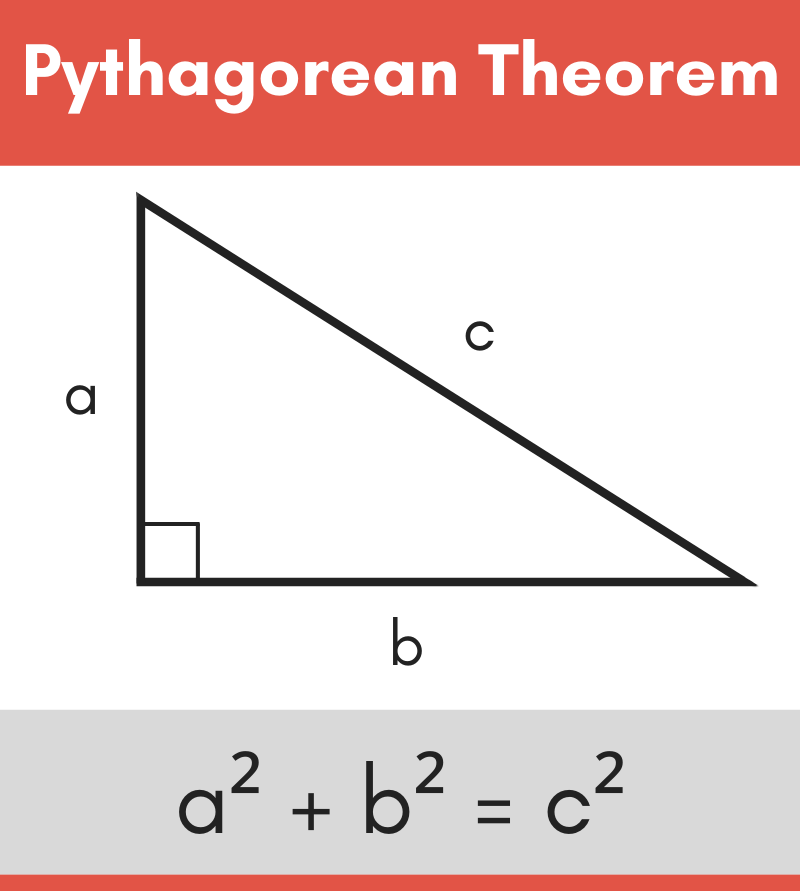
An Introduction To Pythagoras Theorem SyedLearns

pythagorean theorem art Google Search Mathematics art, Math art

Pythagorean theorem, original drawing on prepared paper Pythagorean

Spiral of Pythagoras year 4 Math art projects, Math art, Spiral drawing

How to Make A Spiral from the Pythagorean Theorem Sciencing

How to Prove the Pythagorean Theorem 10 Steps (with Pictures)
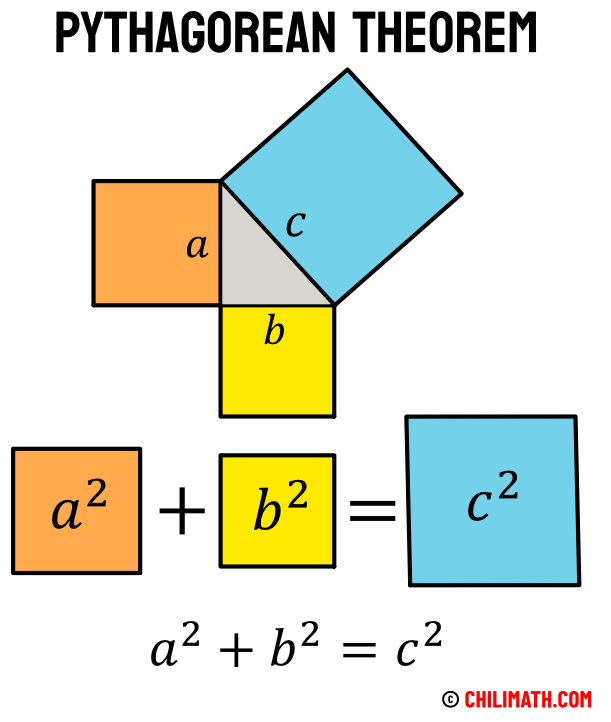
Pythagorean Theorem Definition, Formula & Examples ChiliMath
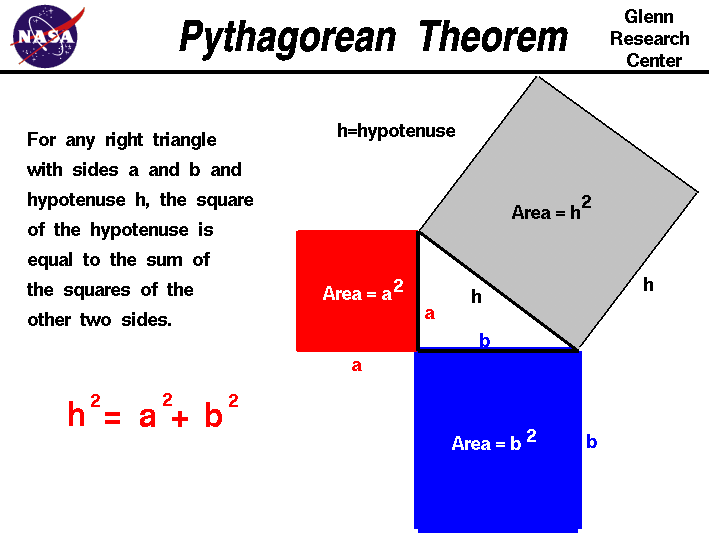
Pythagorean Theorem
Discover How To Find The Length Of An Edge In A 3D Shape Using The Pythagorean Theorem!
If We Plot These Points On A Grid And Connect Them, They Make A Diagonal Line.
Square Root Of Both Sides:
Example \ (\Pageindex {5}\) Example \ (\Pageindex {6}\) Example \ (\Pageindex {8}\) The Distance Formula.
Related Post: